Pascal’s Triangle

2016-03-26, post № 111

mathematics, programming, Pygame, Python, #crown, #generate, #sequence

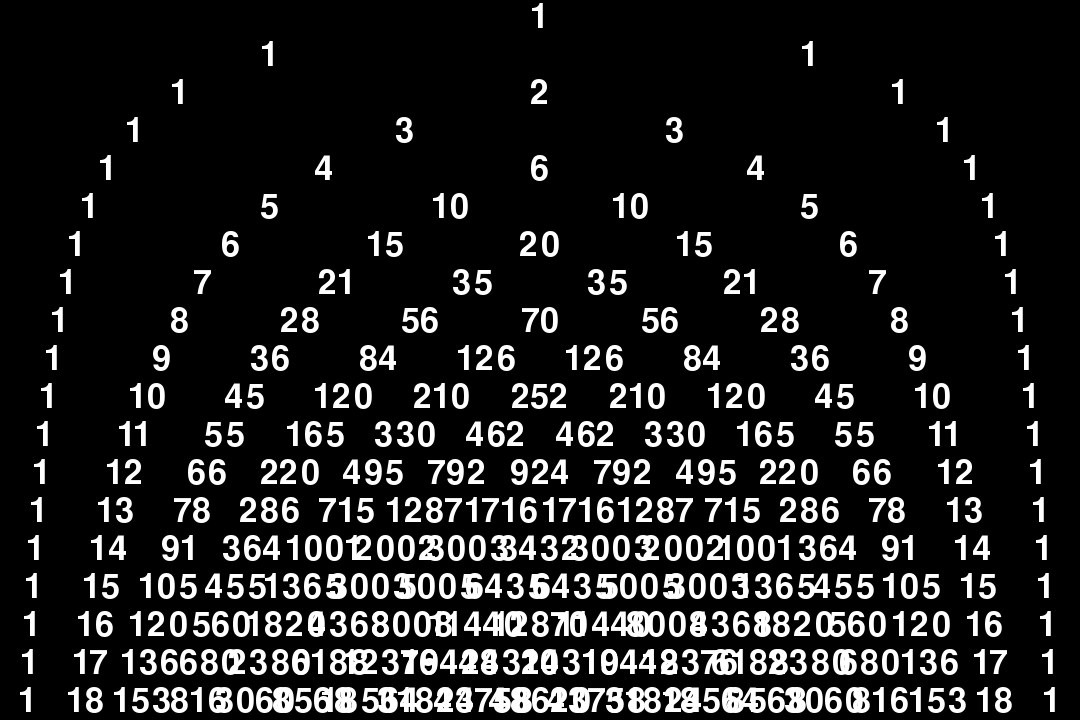
Pascal’s triangle is an interesting mathematical sequence. It is often written as a triangle, starting with $\{1\}$ , then $\{1,1\}$ . To generate the next row, you add the two numbers above to form another one. So the next row in the sequence is $\{1,2,1\}$ then $\{1,3,3,1\}$ , $\{1,4,6,4,1\}$ and so on (sequence A007318 in OEIS).

One interesting property of Pascal’s triangle is the generation of binomials.
To calculate $(a+b)^4$ , you can look at the 𝟦-th row (listed above and when starting to count at 𝟢) and determine

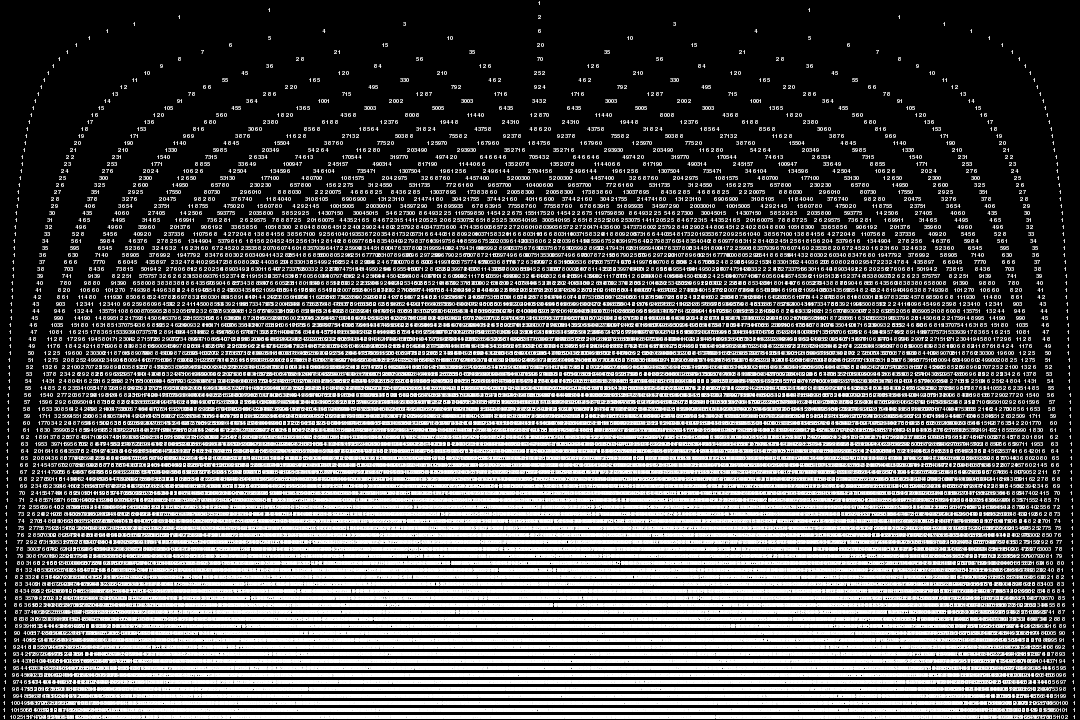
$(a+b)^4=(1\cdot a^4\cdot b^0)+(4\cdot a^3\cdot b^1)+(6\cdot a^2\cdot b^2)+(4\cdot a^1\cdot b^3)+(1\cdot a^0\cdot b^4)$

This program generates Pascal’s sequence in a rather unusual shape, looking a bit like a crown.

To get more information about Pascal’s triangle, check out this Wikipedia entry.

Controls

‘F1’ advances the sequence,
‘Space’ takes a screenshot.

Source code: pascals-triangle.py

Extra assets: pascals-triangle-3-fontsize-30.png, pascals-triangle_fail.py, pascals-triangle_pascal.py, pascals-triangle_latex.snippet.html

^[1]

Footnotes

▲ In pascals-triangle_pascal.py there is a typo: ‘sedonds’ should say ‘seconds’.